Diffraction & Interference

  
   

Imagine plane wavefronts moving toward a wall with a hole in it. If the hole is very wide, the waves coming through the hole will be mostly flat, but will curve outward (i.e. diverge) slightly.

The spreading out of waves when they pass an obstacle is called diffraction. Smaller holes cause waves to diffract more. If the hole is small, the waves coming through the hole will spread out (diverge) again, as if the hole were a point source of waves, like a rock thrown into a pond.

What counts as "small" depends on the wavelength. If the hole is smaller than the wavelength, then the wavefronts coming out of the hole will be circular. Therefore, longer wavelengths diffract more than shorter wavelengths.

Diffraction happens with all kinds of waves, including ocean waves, sound and light. Here's an aerial photo of ocean waves diffracting as they pass through a gap in a causeway.

Photo © Exploratorium, www.exploratorium.edu. Some rights reserved. This work is licensed under creativecommons.org/licenses/by-nc-sa/3.0/us/ 

 

Activities & Practice
to do as you read

1. Play with this Wave Interference simulation, created by the PhET team at the University of Colorado. Some things to try, for each type of wave: put in a one-slit barrier, change the wavelength, change the width of the slit. What variations cause the most diffraction (wave spreading out the most). Then try putting in a two-slit barrier. Again vary the wavelength, and also the slit separation. On the Light tab, hit the "show screen" button and notice the alternating bright and dark areas on the screen.

2. Typically, you can hear sound from around a corner, but you can't see around a corner. Why?

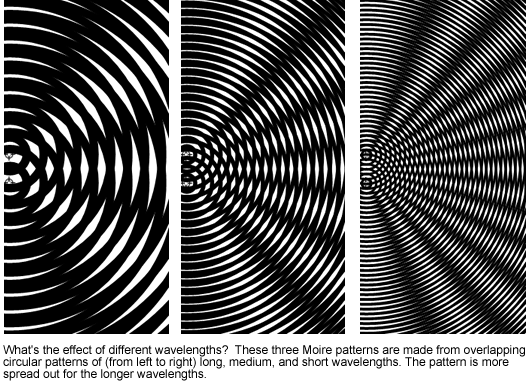
If plane waves come to a barrier that has two holes, the diffracted waves emerging from those holes will overlap and interfere with each other. The resulting pattern of alternating constructive and destructive interference is reminiscent of Moiré patterns. As shown in the following graphics, the pattern is more spread out when (1) the holes are closer together, or (2) the waves are of longer wavelengths.


 3. Face a blank, light-colored wall. Hold one hand flat, right in front of your eye, touching your nose. Keep the index finger and middle finger next to each other, almost touching but with the thinnest slit of light between them. Carefully peer through that thin slit. You will see a series of dark bands in that gap, parallel to your fingers. These are zones of destructive interference.

The double-slit interference equation, as derived in class, is

The slit-to-slit distance is d, and lambda is the wavelength.

4. Play with this applet: http://www.walter-fendt.de/ph14e/doubleslit.htm

NOTE: the author of this webpage uses k to count the order of the bright spots ("maxima"), whereas I use n.

Additional Activities & Practice

5. The diffraction grating we use in class has a spacing of 750 slits per mm. That is, the distance from one slit to the next (d) is 1/750th of a mm. (a) If you shine a violet laser (wavelength 405 nm) through the grating, at what angle do you get the "first-order" (n=1) bright spot? (NOTE: you will need to use the inverse-sine ("arcsine") function of your calculator.) At what angle is the second-order (n=2) bright spot? (b) If you shine the laser perpendicular to a screen and through the grating located 2.0 meters from the screen, how many cm from the central bright spot (n=0) will the first-order spot be?

6. Design an experiment to determine the wavelength of a laser, using a grating with known spacing (d), a wall and some metersticks. Derive an equation for the wavelength, and then describe the procedure you would follow.

  

 

  

 

HTML Comment Box is loading comments...