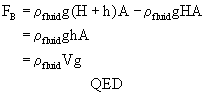Buoyancy
to do as you read
Archimedes, a Greek mathematician and resident of the Greek colony Syracuse on the island of Sicily in the 3rd century BC, is credited with discovering how to calculate the strength of the buoyant force. Archimedes' Principle states that the buoyant force pushing upwards on an object is equal to the weight of fluid displaced by the object.
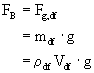
Archimedes (287-212 BC)
Why does buoyancy happen? Recall from our discussion of pressure that the deeper you are in a fluid, the greater the pressure. A consequence of that is the pressure pushing (upwards) on the bottom of an object is greater than the pressure pushing (downwards) on the top of the object. Let's try to derive how strong the resultant force would be...
Proof of Archimedes' Principle For the sake of simplicity, consider a block submerged in water. The area of the bottom of the block is A, the same as the area of the top of the block. The height of the block is h and the depth from the surface of the fluid to the top of the block is H. Fluid pressure will push downwards on the top of the block, and that force will be
The force pressing up on the bottom of the block will be
The resultant force of these two is the buoyant force, FB. Because they are pointing in opposite directions, we subtract in order to find the resultant:
If you'd like to see that proof in video form, click this thumbnail...
1. Archimedes was a fascinating character. You can learn more about him from the Wikipedia article "Archimedes". Read at least the introduction (above the table of contents) and the section titled "The Golden Crown".
2. Play with this buoyancy applet at McGraw-Hill. Put the box/boat in the water, then add some "cargo". What happens to the boat as you add weight? What happens to the boat when, for the same weight of cargo, you change the fluid to mercury? To alcohol?
Play also with this other buoyancy applet, from W. Fendt. What happens to the scale reading (the "measured force") when the block is submerged in the water?
EXAMPLE Calculate the buoyant force acting on an 8-kilogram block that is 10 cm wide, 20 cm deep, and 30 cm high, held completely submerged in a pool of water? When released, does the block sink to the bottom or float to the surface? If it floats, what percentage of it is sticking out of the water? If it sinks, what is the normal force, FN with which it sits on the bottom of the pool?
SOLUTION The volume of the block is 0.006 m³. The block is completely under the water, so the volume of displaced water is equal to the volume of the whole block. The weight of the displaced water is...
So the buoyancy is pushing upward with a force of 59N. Gravity, on the other hand is pulling down with a force of
The block, therefore, will sink to the bottom and come to a rest there, supported by the floor with normal force of
78N - 59N = 19N
3. (a) What is the buoyant force on a one-cubic-meter block of styrofoam, completely submerged in water? (b) What is the buoyant force on a one-cubic-meter block of concrete, also completely submerged in water? (c) Left alone, the syrofoam block will float and the concrete block will sink. Why the difference between the two?
Does It Float or Does It Sink? The buoyant force is always upwards. Imagine you have an object that is fully submerged under a fluid. The object will displace a volume of fluid equal to its own total volume, so the buoyant force would be ρfluidVg. Besides the upwards buoyant force, what other force is pulling on the object? Yes, gravity, the weight of the object. The object's weight is equal to mg, which is equal to ρobjectVg.
So, if the density of the object is greater than the density of the fluid, the weight will be more than the buoyancy, the net force will be downwards, and the object will sink. This is called negative buoyancy, and the object will sink until it comes to a rest on the bottom of the pool, lake, ocean, container, or whatever is containing the fluid. The object will sit on the bottom, but the normal force, FN, that it exerts on the bottom will be less than it would be without the buoyancy from the fluid.. If the object's density is less than the
If, on the other hand, the density of the object is less than that of the fluid, it has positive buoyancy, the net force will be upwards and the object will rise until it reaches the surface of the water. It will float, with some of the object sticking above the surface. It will sit just enough in the fluid so the weight of the displaced fluid equals its own weight. The lesser the density of the object, the more of it will sit above the surface of the fluid.
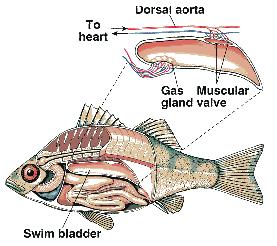
If the density of the object is exactly the same as the surrounding fluid, Fg=FB and therefore FNET=0. The object will not accelerate downward or upwards. This is neutral buoyancy. Most fish have swim bladders into which they can release gas, changing their overall volume and density to precisely match the density of the surrounding water. (The density of the water varies some, depending on salt content, temperature and pressure.) SCUBA divers do the same thing. Divers wear a buoyancy compensator (also known as a "BC"), an inflatable jacket into which they can pump air directly from the tank, or from the diver's own exhalation. By adjusting their overall density to match the density of the surrounding water, fish and SCUBA divers don't have to exert effort to keep from sinking or rising.


4. Six objects (A-F) are in a liquid, as shown is this side view. None of them are moving.
Arrange them in order of density, from lowest to highest.
5. Water ice has a density of 0.91 g/cm³, so it will float in liquid water. Imagine you have a cube of ice, 10 cm on a side. (a) What is the cube's weight? (b) What volume of liquid water must be displaced in order to support the floating cube? (c) What percentage of the cube volume is under the surface of the water?
6. You have a block of a mystery material, 12 cm long, 11 cm wide and 3.5 cm thick. Its mass is 1155 grams.
(a) What is its density?
(b) Will it float in a tank of water, or sink?
(c) If it floats, what percentage of the object will be sticking out above the water? If it sinks, what will be the normal force it presses against the bottom of the tank?
(d) Repeat parts b and c, only instead of water, the tank is full of mercury.
Look at the only after making your best attempt at the problems.
Buoyancy in the Atmosphere
Notice that in all the above discussion, I always used the word "fluid" rather than "liquid" when talking about the stuff the object is immersed in. Fluid refers to anything that flows, i.e. to both liquids and gases. If an object is immersed in a gas, it experiences an upward buoyant force given by Archimedes' Principle, just like for a liquid. Hot-air balloons, of course, are a good demonstration of this, as are the smaller helium-filled balloons typically seen at birthday parties. Both types of balloons are filled with a low-density gas (hot air and helium, respectively) so that overall, even taking the weight of the fabric or plastic into account, the filled balloon is less dense than the surrounding air.
Way back, hydrogen gas was often used for balloons and airships: it's much less dense than hot air, and cheaper than helium. It is terribly combustible, however, and that's a big downside, as the passengers of the Hindenburg discovered on 6 May 1937.
There is buoyancy acting on you right now. Your body displaces an equal volume of air, so there is an upward force of buoyancy acting on you. Of course, it's not enough to support your entire weight, but when you stand on a bathroom scale, the reading is a little bit less than it would be if there were no air in the room.
Is there buoyancy in Space? Recall that the buoyant force is equal to the weight of displaced fluid. If there is no gravity, or if you are falling so it feels as if there is no gravity, then there will be no buoyancy. This would be the case aboard the Vomit Comet, or in orbit. Here's a movie taken aboard the International Space Station showing an air bubble being injected into a floating blob of water with a hypodermic needle. The air bubble is perfectly happy staying in the middle. Without an "up", it wouldn't know which way to rise!
Additional Activities & Practice
7. A standard basketball (mass = 624 grams; 24.3 cm in diameter) is held fully under water. Calculate the buoyant force and weight. When released, does the ball sink to the bottom or float to the surface? If it floats, what percentage of it is sticking out of the water? If it sinks, what is the normal force, FN with which it sits on the bottom of the pool? SOLUTION VIDEO
8. Ten-pin bowling balls are 21.8 cm in diameter.
(a) What is the volume of one of these bowling balls?
(b) What is the buoyant force acting on a bowling ball, if it is completely under water?
(c) What would a bowling ball's mass have to be, in order for it to have the same density as water? (What is that, expressed as weight, in pounds? Remember, 1 kg on Earth weighs 2.2 lbs.)
(d) Bowling balls come in a range of masses or weights, from 6 pounds (2.7 kg) to 16 pounds (7.2 kg). The lighter ones float; the heavier ones sink. If a 6-pound ball is floating, what percentage of its volume is sticking up out of the water?
9. A boat that weighs 120,000 Newtons is floating peacefully on a lake. The boat is like a box, 7 meters long, 3 meters wide, and 2 meters tall.
(a) MULTIPLE CHOICE: The buoyant force acting on the boat is _____.
A. 120,000 N B. more than 120,000 N C. less than 120,000N(b) What volume of water must be displaced in order to hold the boat up?
(c) How much (in meters) of the 2-meter height of the boat is below the surface of the lake?
(d) MULTIPLE-CHOICE: If the same boat were floating in the ocean instead of a lake, it would sit...
A. A little bit lower in the water
B. A little bit higher
C. Just the same as it does on the lake.
10. Is a spherical helium balloon 5.0 meters in diameter big enough to lift a person? The density of air near the ground is 1.25 kg/m3. The density of helium at one atmosphere pressure is 0.18 kg/m3.
(a) What is the volume of the balloon?
(b) What is the buoyant force?
(c) What is the weight of the helium in the balloon?
(d) What is the net force? Assume the weight of the balloon itself (the plastic bag that holds the helium) is insignificant.
(e) Is that net force enough to lift a person? Assume the person is 50 kg in mass.